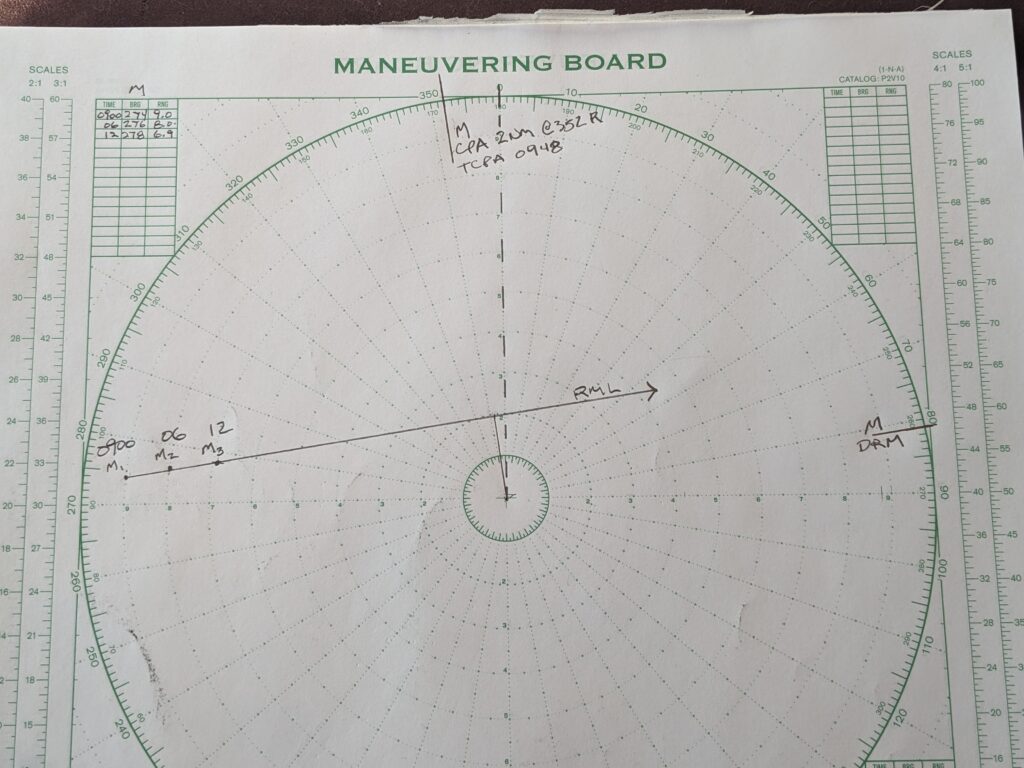
In the CPA – Closest Point of Approach lesson we discussed general plotting concepts of another vessel’s relative movement using Distance and Bearing along various time intervals to derive a solution as to how close the other vessel will approach if your course and speed and their course and speed remain unchanged.
The example used developed an RML between “you” and vessel “M” of 081R, with a CPA of 2 miles at 352R. TCPA was found to be 0948, 48 minutes after point of first contact.
Obviously, there is no risk of collision in this case, however, some additional information may be developed that will be useful.
Relative Motion to True Motion
The solution thus far only provides information regarding the relative movement of the other vessel compared to you, not its true course and speed. There are times the true course and speed of the vessel are needed. A vector triangle is the answer.
Head Up vs North Up
At this point it is critical to determine if your display is set to head up or north up. The steps are similar with the different views, however, there are some important differences. This exercise will assume you are operating in head up mode.
Three Vectors Make a Solution
- Own Ship Course and Speed Vector
- Relative Motion Course and Speed Vector (RML)
- Other Ship True Course and Speed Vector
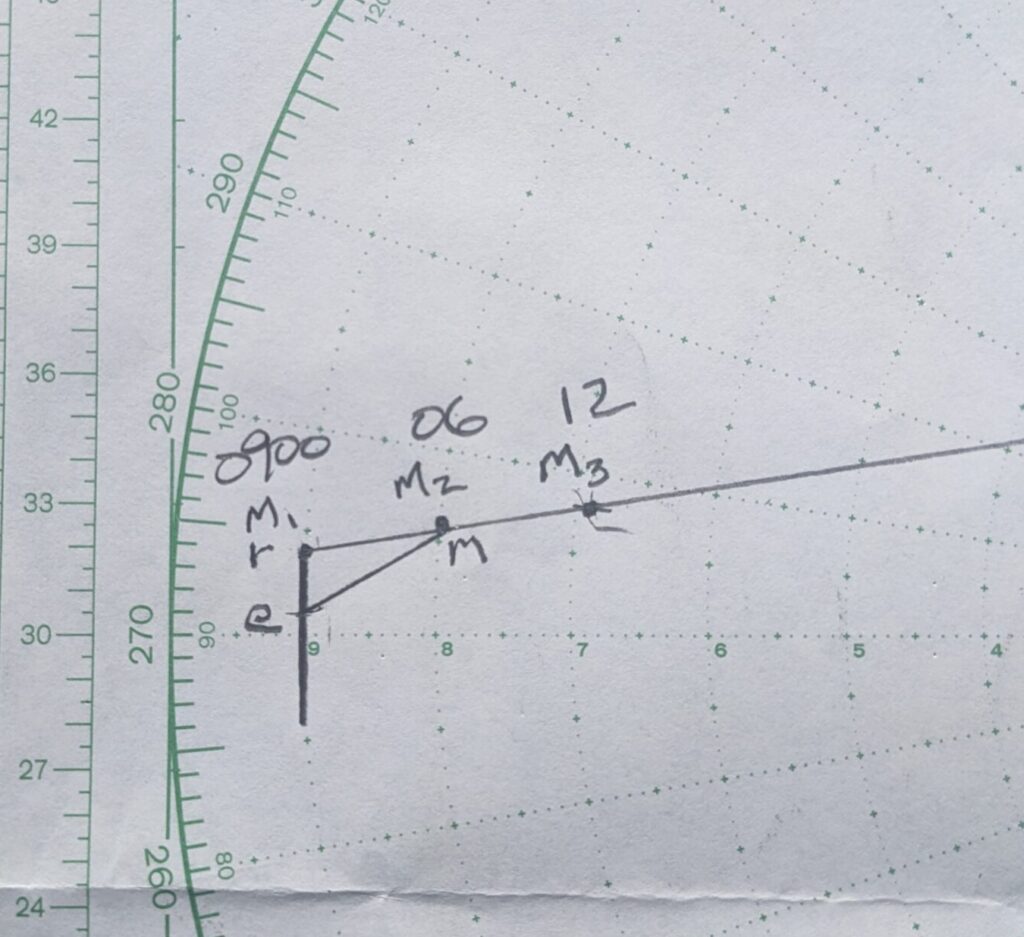
To determine true motion, start by plotting what you know. You know your own course and speed vector, and you know the relative course and speed vector.
Since you want to plot what your vessel did during the time you were tracking the other vessel, begin at point M1 and plot your course and speed backward in time. Remember that you are in head up mode, meaning you will plot your course backward from your head up course of 000 a distance equal to your speed. Assuming a speed of 5 knots, over a six minute time period, you would travel .5 miles.
Three new labels and three new combinations of those labels need to be introduced at this time.
other ship true course and speed “em”
Vector Triangle Plot Labels
- e … The origin of any ship’s true (course-speed) vector; fixed with respect to the earth. This label will represent the origin of your ship’s course and speed as well as the other vessel’s true course and speed.
- r … The end of own ship’s true (course-speed) vector, er; and/or the origin of the relative (DRM-SRM) vector, rm.
- m … The end of other ship’s true (course-speed) vector, em; and/ or the end of the relative (DRM-SRM) vector, rm.
Combining the e, r, and m labels on the plot results in the following vectors.
- er – Own ship’s true (course-speed) vector
- em – Other ship’s true (course-speed) vector
- rm – The relative (DRM-SRM) vector; always in the direction of M1→ M2→ M3 …
Labeling the Vector Triangle
Point M1 will now also be known as “r”
Point M2 will now also be known as “m”
The beginning of your course is known as “e”
Line em is the other vessel’s true course (or vessel aspect) and speed. In this case, a bearing 060 @ 12 knots.
Aspect
At its CPA, you should be looking at the starboard “green light” side of the vessel which will be oriented 60∘ from head on and can be stated at a “green 60∘” aspect. This becomes important when determining the burdened vessel’s responsibilities.
Course and Speed to Avoid a Passing Vessel
The previous example did not result in a possible collision course or the requirement to maneuver to avoid. Let’s try another now.
Your vessel is on a course of 000∘ with a speed of 6 knots. The radar is set to head up mode. At 1730 another vessel (M) is observed at 060R with a range of 9 miles. At 1736, the bearing is 060R and the range has reduced to 7.6 miles. Use a mo board to determine the following:
- What is the DRM, SRM, CPA, and TCPA?
- What is the true course and speed of M?
- What is the aspect?
Assuming you are the burdened vessel, we will calculate a course to steer to allow M to pass 1.5 miles ahead of our ship, with us executing the maneuver at a range of 5 miles.
New Relative Motion Line (NRML)
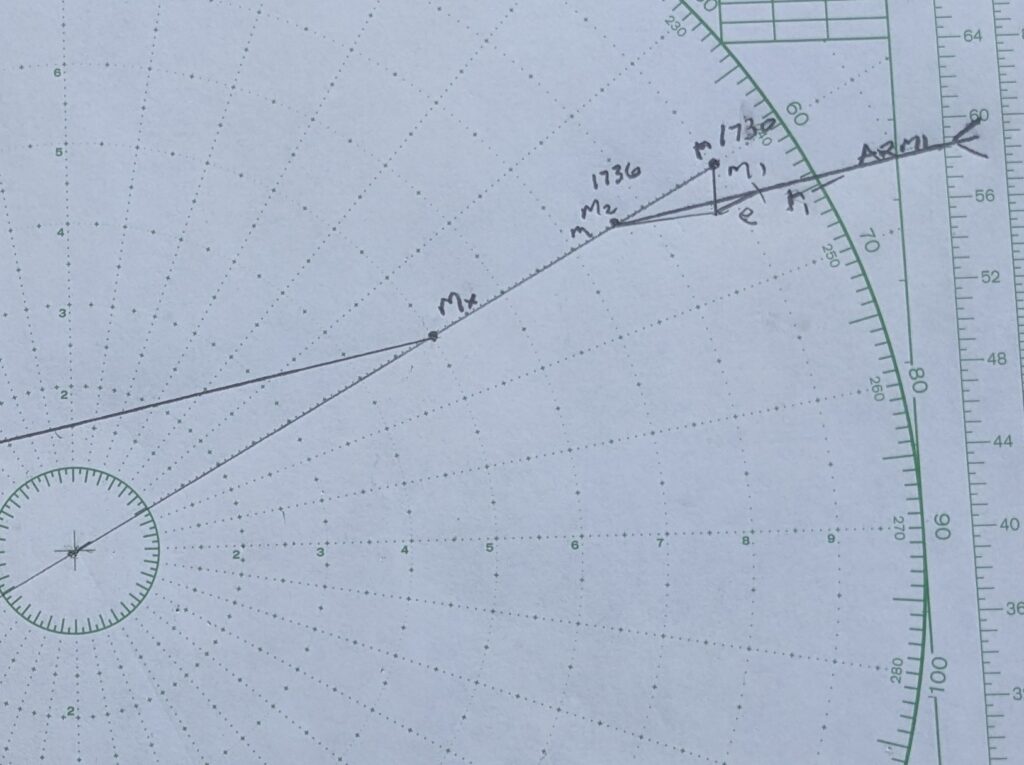
Start by indicating the spot to make your turn (Mx) at a range of 5 miles along the RML. Draw in a new relative motion line (NRML) beginning at Mx and just touching the 1.5 mile range ring.

and 1.5 Mile CPA
Start by indicating the spot (Mx) to make your turn at a range of 5 miles along the RML. Draw in a new relative motion line (NRML) beginning at Mx and just touching the tangent of the 1.5 mile range ring.
The NRML here is 258R, and needs to be labeled.
Move, or advance, the NRML until it ends at point M2. This new line becomes ARML.
Course to Steer
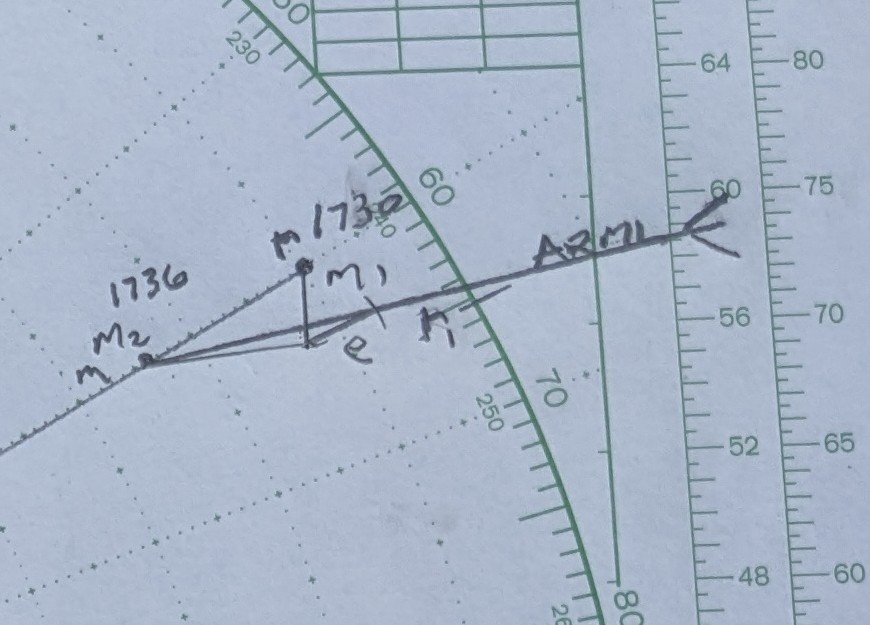
Set dividers to the distance of er (in this case, 6 miles) and strike an arc centered on e that intersects ARML. The intersection becomes r1 (aka r prime).
Your new course to steer is 065. According to the original SRM, expect to arrive at Mx at 1747. On the new course, CPA will be 1.5 miles, 11 degrees off the port bow (349R), with a TCPA of 1803 (r1m provides the a new SRM of 18 knots). Aspect will be red 102∘ (nearly off the other vessel’s port beam).
A Second Method
A second method of finding the same solution is available that many find more convenient as well as more accurate. Instead of completing the true course and speed and course to steer vector triangles using points M1 and M2, move them to the center of the mo board.
True Course and Speed Vector Triangle
One major difference exists. Instead of plotting “own ship” course to end at m, plot er beginning at the center and going in the direction of travel a distance equal to own ship speed. The RML line will then start at r and go towards m at a distance equal to SRM. “em” can now be added to the triangle. In the image, the unhighlighted pencil lines describe true course and speed vector triangle.
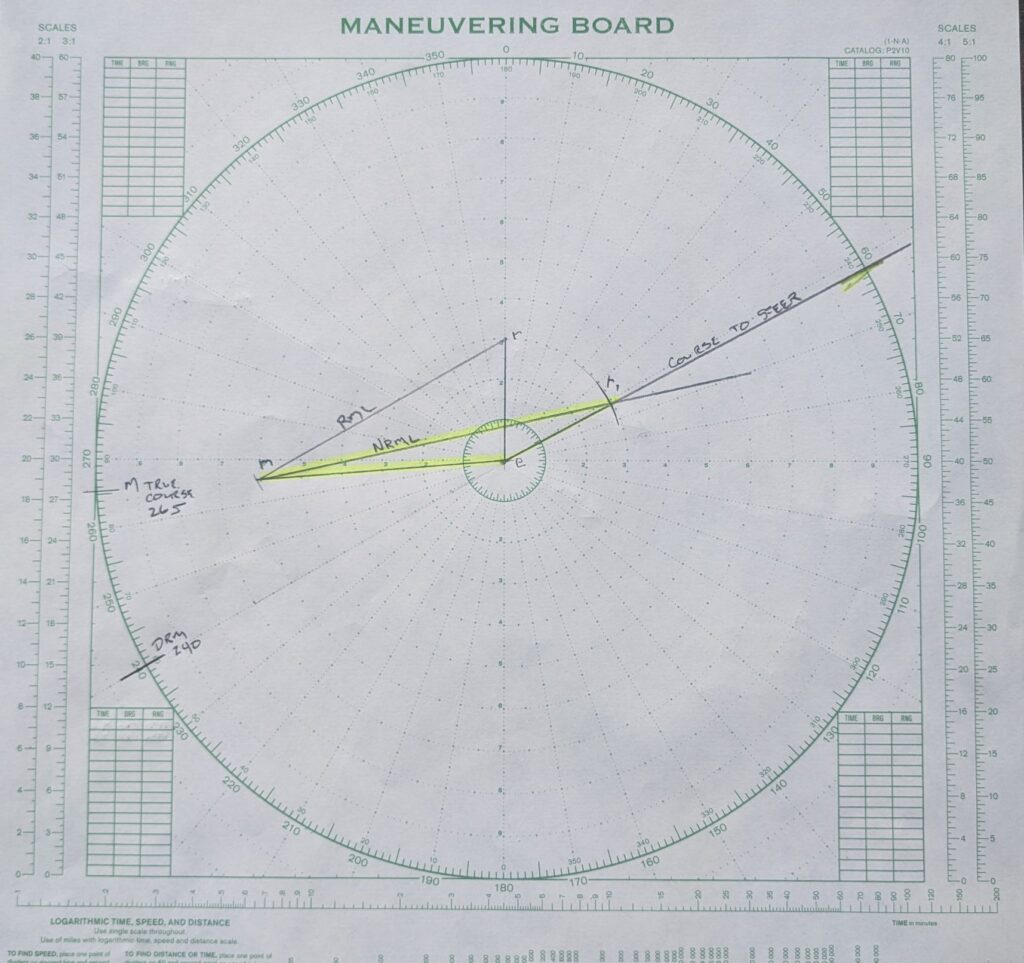
Course To Steer Vector Triangle
The course to steer vector triangle can now be completed. There is one constant between the true course and speed triangle and the course to steer triangle. Vessel M has a course of 265 and a speed of 12 knots. The em vector will not change. We also know NRML which was found using the original plot. Add the NRML to the plot, ending at m.
Set dividers to the distance of er and scribe an arc that crosses NRML. This is r prime. Your new course to steer is er1 (062). In the image, the course to steer vector triangle has been highlighted in yellow.
You may notice, the first method gave a solution of 065 while the second method provided 062. A small difference which will be negligible in practice. I tend to prefer the second method because the size of the triangles tends to provide greater accuracy.
Course to Steer Problem Answers
